Time dependent concentration in a first order reversible reaction in a batch reactor
Posted February 05, 2013 at 09:00 AM | categories: ode | tags: reaction engineering
Updated March 06, 2013 at 04:28 PM
Given this reaction \(A \rightleftharpoons B\), with these rate laws:
forward rate law: \(-r_a = k_1 C_A\)
backward rate law: \(-r_b = k_{-1} C_B\)
plot the concentration of A vs. time. This example illustrates a set of coupled first order ODES.
from scipy.integrate import odeint import numpy as np def myode(C, t): # ra = -k1*Ca # rb = -k_1*Cb # net rate for production of A: ra - rb # net rate for production of B: -ra + rb k1 = 1 # 1/min; k_1 = 0.5 # 1/min; Ca = C[0] Cb = C[1] ra = -k1 * Ca rb = -k_1 * Cb dCadt = ra - rb dCbdt = -ra + rb dCdt = [dCadt, dCbdt] return dCdt tspan = np.linspace(0, 5) init = [1, 0] # mol/L C = odeint(myode, init, tspan) Ca = C[:,0] Cb = C[:,1] import matplotlib.pyplot as plt plt.plot(tspan, Ca, tspan, Cb) plt.xlabel('Time (min)') plt.ylabel('C (mol/L)') plt.legend(['$C_A$', '$C_B$']) plt.savefig('images/reversible-batch.png')
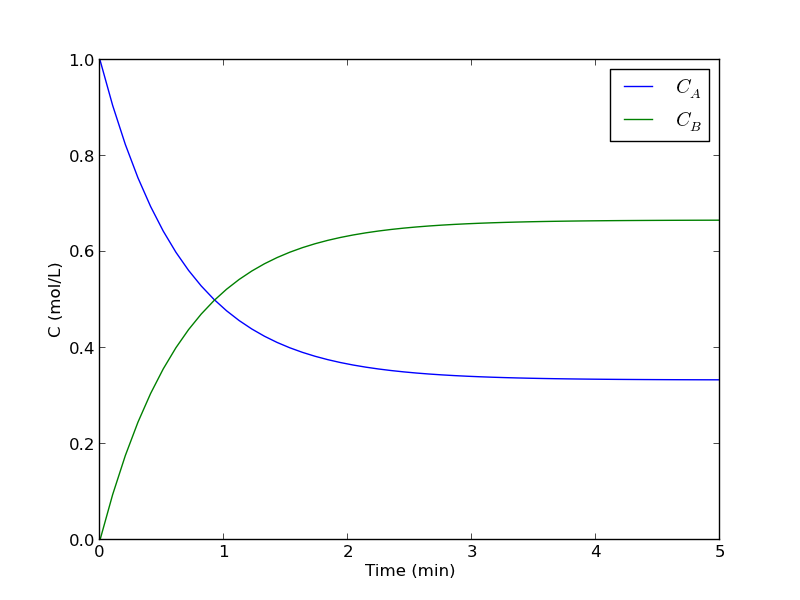
That is it. The main difference between this and Matlab is the order of arguments in odeint is different, and the ode function has differently ordered arguments.
Copyright (C) 2013 by John Kitchin. See the License for information about copying.